[Home] [Contents][Figures] [Search] [Help]
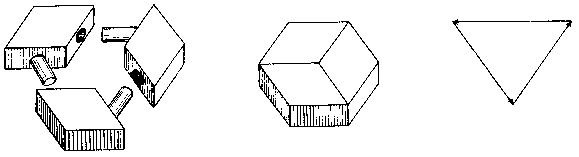
Figure 125 shows a very simple amusement consisting of three identical rhombic blocks with pins and holes. Note that the only way it can be assembled is to start all three pins into the holes simultaneously and bring all three blocks together. This model is a simple example of a coordinate-motion puzzle. Such puzzles cannot be assembled sequentially, but rather at some stage of assembly they require the simultaneous manipulation of three or more pieces or groups of pieces. Unlike this simple example, such puzzles can be very baffling. One such puzzle has already been included surreptitiously in a previous chapter without being identified as such!
Fig. 125
This simple novelty (Fig. 126) is a practical example of a coordinate-motion puzzle in three dimensions. Each of the six identical puzzle pieces is made up of a right-triangular prism center block to which are attached a pair of rhomboid-prism end blocks. They assemble with no great mystery to form a hollow box, but if they are accurately made, some dexterity is required to get all six pieces aligned exactly right and mutually engaged. Once assembled, by holding on to opposite pieces, the puzzle can be made to expand almost to the point of collapse and then to shrink back together again. It is more of an amusement than a puzzle.
Fig. 126
The Rosebud Puzzle consists of six pieces (Fig. 127a), of which three are identical and the other three are their reflexive pairs, except that one piece has a small hole for a removable pin. This puzzle has two symmetrical solutions, one of which (Fig. 127b) is decidedly more interesting than the other. Assembling it in the preferred solution requires the simultaneous manipulation of all six pieces in a manner that to begin with is not at all obvious and furthermore requires dexterity, patience, and some clever tricks.
Fig. 127a
Fig. 127b
A few of these puzzles were produced some years ago and sold unassembled. After sufficient time had elapsed and almost none had been solved, the customers were given the opportunity to purchase (for an outrageous price!) an assembly jig and directions. With these, it is easy. Without the jig, it can be done with patience, using tape and rubber bands. Without such aids, it has been done but borders on the impossible.
The reward for going to all this trouble is to discover the amazing mechanical action of the assembled puzzle. By placing three fingers of each hand in just the right places, it can be made to expand in a most fascinating manner, rather like the petals of a blooming flower (Fig. 127c). This effect is further enhanced by making the blocks of colorful woods with symmetrically matched grain. Then shift all six fingers slightly and the Rosebud closes again. By inserting the pin into the hole after assembly, one can play with the puzzle in this manner with no worry that it will fly apart.
Fig. 127c
Standard building blocks are used in the construction of the puzzle pieces, as indicated. In order to be entirely satisfactory, they must be made very accurately with a smooth sliding action and must be strong.
The mechanical action of a coordinate-motion puzzle can be analyzed and explained using a vector diagram. For instance, in the first example shown, if the three rhombic blocks are A, B, and C, their relative motions are vectors AB, BC, and CA, which must form a closed loop and add up to zero - in this case by an equilateral triangle. The vector diagram of the Expanding Box Puzzle is an octahedron, and for the Rosebud Puzzle it is a triangular antiprism. The vector diagram for a coordinate-motion puzzle cannot be rectilinear. Why?
Although it is conceivable that one might design a coordinate-motion puzzle by studying vector diagrams, most such designs are just stumbled upon. The vector diagrams are presented here mostly as a curiosity.
| ©1990-2012 by Stewart T. Coffin For questions or comments regarding this site, contact the chief metagrobologist: |